Connecting active and passive seismic to describe geomechanical rock properties

The reflection seismic method has its origins in the Earthquake Seismology studies of the nature and types of elastic seismic waves released from an earthquake source. Observations of these waves at the earth’s surface, have led to an understanding of the theoretical basis for elastic compressional P-wave and shear S-wave propagation deep within the earth. Models based on this knowledge reveal information about the structure and geomechanical rock properties of the earth that would have been totally unattainable by any other means. Inverting P-wave and S-wave wave arrival times, has predicted the presence of a lithosphere that is sufficiently brittle to act as the focal region for earthquakes. A crowning success of these studies has established an outer core underlying the lithosphere that supports no S-waves, from which it is deduced to be molten. However, a less well known fact is that the outer core has a sustainably lower P-wave velocity than the lithosphere. This enigmatic observation can be understood from the physics used to derive propagation velocity, which combines Hooke’s law and the elastic wave equation to form the basis for these two seismological methods, as follows.

Hooke’s law for uniaxial normal compressive stress can be written as:

σ(z)_norm = λθ+2μe(z)

where:

σ(z)_norm is the uniaxial normal stress in the z direction θ is the fractional volume change or cubic dilatation in x, y and z directions

e(z) is the normal strain or fractional length change in the z direction =

And for the uniaxial shear stress:

σ(z)_shear = μe(z)_shear

where:

σ(z)_shear is the uniaxial shear stress in the z direction

e(z) is the shear strain or fractional length dux change perpendicular to the z direction =

The respective wave equations that govern the propagation of P-waves and S-waves describe attributes of the propagating medium by including Hooke’s law.

The P-wave propagation of the dilatation θ term (seen in the compressional version of Hooke’s law above) can be written as:

where ρ is density, ∇ is the spatial derivative operator and θ is cubic dialatation or volume change and the S-wave propagation of the shear displacement term u_x (seen in the shear version of Hooke’s law above) can be written as:

where ∇ x U is the rotational displacement or shear strain gradient without a volume change θ

These basic seismological equations above are not presented for some arbitrary intellectual reason, but instead they emphasize that the moduli involved at the basic level of geophysical description of seismic phenomena are the intuitively simple, orthogonal Lamé parameters of rigidity Mu (μ) and incompressibility Lambda (λ) and to a lesser extent the bound (in situ) uniaxial P-wave propagation modulus λ+2μ that is nameless (Goodway et al 1997, 2001, 2006).

Conventional seismic techniques successfully utilize controlled sources of seismic energy to propagate primarily P-waves into the earth in order to reveal its structure through reflection imaging. As a result there are major differences between reflection seismic and Earthquake Seismology, despite sharing common wave propagation physics at their widely disparate scales of investigation. Earthquake Seismology involves curved or diving ray transmission through the Earths interior that gives rise to arrival time-distance plots that have the form of a hyperbolic sine function or refraction, due to a linear velocity gradient with depth. By contrast the seismic reflection NMO curve is a result of essentially reflected straight rays that produce hyperbolic arrival time-distance plots as a function of RMS velocity, and give rise to reflectivity series and AVO. Since arrival timedistance observations are the most obvious mode of analysis, inversion for the velocity structure of the subsurface is also another common aspect of both methods. However just obtaining values for the velocity structure of the earth, either Pwave or S-wave, reveals only limited information about the propagating medium’s physical properties. To obtain insight into the medium’s properties contained in its propagation velocity, requires a plane wave solution for displacement u, of the appropriate wave equation, that has the from of u = A^{eiω(t–x/V)}.

Substituting this solution into the wave equations above results in the representation of velocity in Lamé moduli and density terms. As a result both P-waves and S-waves can now be combined to describe the rock properties of the subsurface. However the ambiguity in using velocity alone to describe the propagating medium still exists, as seen in the following relationships that can be termed as being derived mixtures of the two fundamental moduli λ and μ, and density ρ:

Vp² = (λ + 2μ)/ρ = (κ + (4/3)μ)/ρ, where bulk modulus κ = λ + (2/3)μ and Vs² = μ/ρ

Despite this understanding, standard AVO methods that extract attributes such as intercept and gradient, P-wave and S-wave reflectivity or Vp/Vs velocity and Poisson’s ratio, are yet more ambiguous as they contain more complicated permutations of Lamé moduli λ and μ, or Lamé impedances LambdaRho λρ and MuRho μρ (Goodway 2001).

The bulk modulus kappa (κ) in particular, requires some clarification as it is embedded in the P-wave velocity (Vp) and is generally considered to represent incompressibility, while λ is undefined, being termed as just the first Lamé parameter. However λ is a pure, unambiguous and true measure of incompressibility, as it relates axial and lateral strain to uniaxial stress (as shown by the dilatation term θ in Hooke’s law above). Consequently λ is primarily a longitudinal or normal quantity and therefore orthogonal (i.e. independent) to μ a quantity that relates a transverse shear stress to shear strain. This is not the case for the volumetric bulk modulus κ since it implicitly relates to a measure of shear strain, hence the shear modulus μ, due to the volume’s change in shape resulting from triaxial or hydrostatic stress.

An example of the insight gained by formulating velocities in Lamé moduli terms, can be seen in the Earthquake Seismological observation of the P-wave velocity drop in going from lithosphere to outer core as mentioned above. This can now be easily explained as due to a loss of the rigidity modulus μ in the lithosphere’s P-wave modulus λ+2μ contained in the velocity going from the lithosphere to the molten outer core. This results in a Pwave that must propagate with a lower velocity in the molten outer core as a consequence of being supported by a single λ fluid modulus alone.

The presentation will show how the ambiguities that arise from conventional derived moduli (such as Young’s modulus (E) and Poisson’s ratio (ν)), also permeate through to completions engineering. Understanding both seismology and geomechanics in Lamé moduli terms can be further developed to unify and reveal a deeper physical understanding of both geophysics and engineering. In addition, through application of the original Earthquake Seismology methods, this approach can be shown to better utilize the full potential of the new emerging revolution in microseismic monitoring of hydraulically stimulated fractures; and to extract geomechanical attributes required for improved unconventional tight gas production.

For example, the optimum geomechanical characteristics such as those of the Barnett shale, can be used as an analogue to help guide reflection seismic techniques in identifying the resource potential of similar gas shales. Engineering literature identifies key Barnett shale mechanical properties as requiring high E and low ν that are related empirically in the following linear equation:

ν = 0.452 - 0.0457E.

In order to better understand the significance of E and ν these parameters are converted to the more seismically understandable parameters of λ and μ through the following relationships:

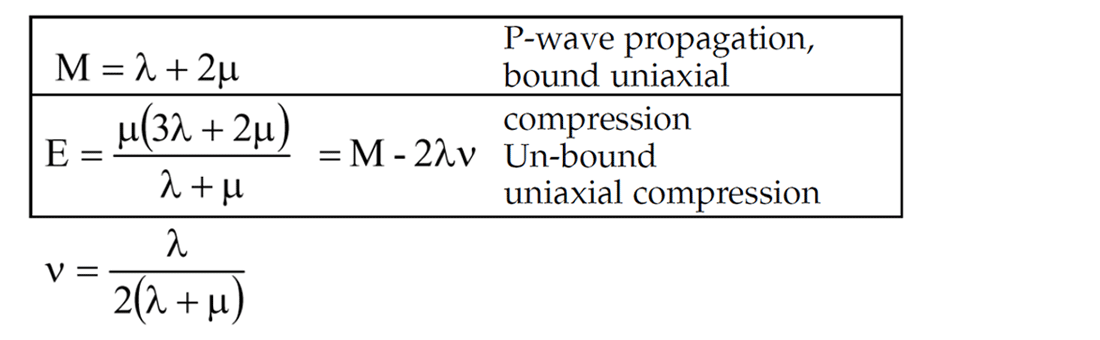
From above E and ν can be related as:

This last expression shows that the inverse linear relation between increasing E to decreasing ν shown for the Barnett shale, is simply the result of an increase in the modulus of rigidity μ. This is yet another case of how the ratio of ambiguous and complicated derived modulus E and ratio ν, have a far simpler and singular representation as the rigidity modulus μ. Transforming this linear relation for the Barnett puts the shale into a brittle region in a λ vs. μ cross-plot with mid range μ and low λ due to its high quartz content.

The presentation will also show how isotropic and azimuthal AVO (AVAZ) measurements can extract lithologic and geomechanical properties from surface seismic data as part of a new Closure Stress mapping method. This method incorporates new attributes from microseismic analysis which are based on a resurgence and combination of the original Earthquake Seismology with the reflection seismic method, to monitor hydraulic fracturing of unconventional tight gas plays.

In conclusion Lamé moduli can be shown to be a unifying link between Earthquake Seismology, reflection seismic, geomechanics, and passive microseismology. Applying this basic principle to many geophysical and engineering problems can add much insight and intuition, resulting in new exploration techniques.