Image Mispositioning in Foothills Seismic Data Due to a Dipping Transversely Isotropic Overburden: Implications from Physical Seismic Modelling Studies

Abstract

Significant errors occur in the position of a target imaged beneath a dipping transversely isotropic (TTI) overburden if the effects of anisotropy are not included during data processing. Using 2-D and 3-D seismic data acquired over scaled physical models, we demonstrate that the imaged position of a target is shifted laterally by a significant distance in the updip direction of the overlying TTI layer. If the 2-D data are depth migrated using a velocity model that gives consistent depth images in the common-offset domain, then the images are too deep by about 3% of the overburden thickness.

Introduction

Many hydrocarbon resource exploration and development plays in the Alberta Foothills involve dipping clastic sequences lying above the target zones. These clastic sequences often contain considerable amounts of shale, which usually possess some degree of intrinsic seismic velocity anisotropy, caused primarily by the orientation of laminations.

We investigate the magnitude of positioning errors in seismically imaged structures by creating physical models with a tilted transversely isotropic layer, that simulates dipping sand/shale sequences, in the overburden. We show on 2-D and 3-D modelling data that the presence of such a TTI overburden above a target reflector gives rise to a significant lateral shift on processed data of the true position of the target if velocity isotropy is assumed during data processing.

The physical model

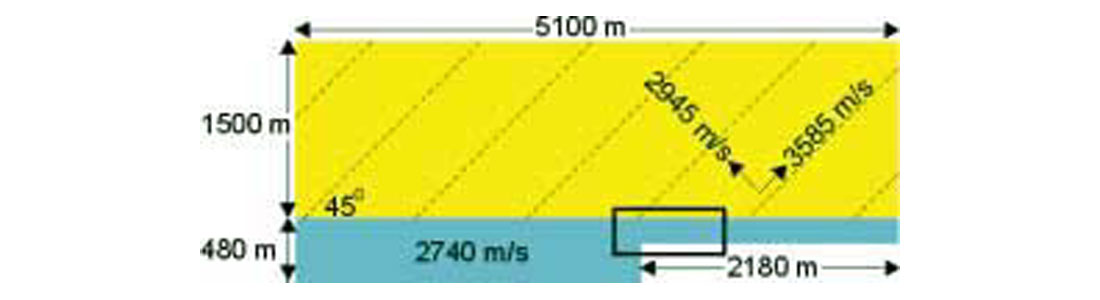
The material used to simulate a TI formation is composed of layers of linen saturated and bonded with a phenolic resin. These thin layers may be regarding as being analogous to fine-scale stratigraphic laminations. The simulated overburden is composed of a rectangular slab of this material, with the TI axis of symmetry dipping at an angle of 45°. The seismic surveys were acquired in the physical modelling laboratory of the Department of Geology and Geophysics at the University of Calgary. In the following discussion, all units of time and distance have been scaled by 10,000 from the model size to real world values. The scaled seismic data generally have a bandwidth of up to 60 Hz, with a central frequency of 20 Hz. For the 2-D survey, the TTI material has measured anisotropy parameters of ε = 0.24 and δ = 0.10 and for the 3-D survey these parameters are ε = 0.296 and δ = 0.198.

2-D survey

For the 2-D survey, a piece of isotropic Plexiglas was affixed to the underside of the TTI block (Figure 1), which has a scaled thickness of 1500 m. This Plexiglas layer has a 90° step in it to simulate a target fault or a reef edge. The isotropic layer is 190 m thick over the upper leg of the step and 480 m thick over the lower leg. A multichannel 2-D survey was acquired with a source station interval of 120 m and a receiver station interval of 20 m. Source-receiver offsets ranged from 240 m to 2120 m; the 240 m source-receiver near offset being caused by the physical limitations of the physical modelling recording system.

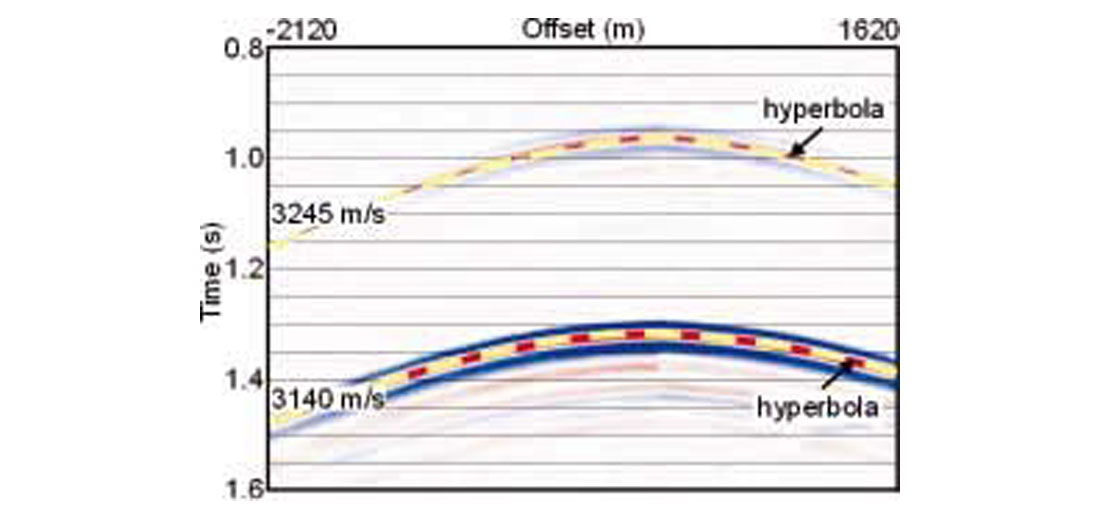
A shot gather (Figure 2) shows that the moveout on the reflectors is very close to hyperbolic, even at the far offsets, and that the moveout velocity falls within the range of the anisotropic material’s velocities. The traveltimes of the reflections exhibit only small departures from hyperbolic moveout. Hence, standard velocity analysis of CMP gathers gives the impression of isotropic data with hyperbolic moveout and yields interval velocities that are within the range of the true velocities of the material. Thus, there is no clear indication on the shot gathers or in the velocity analysis that there might be anisotropy present.

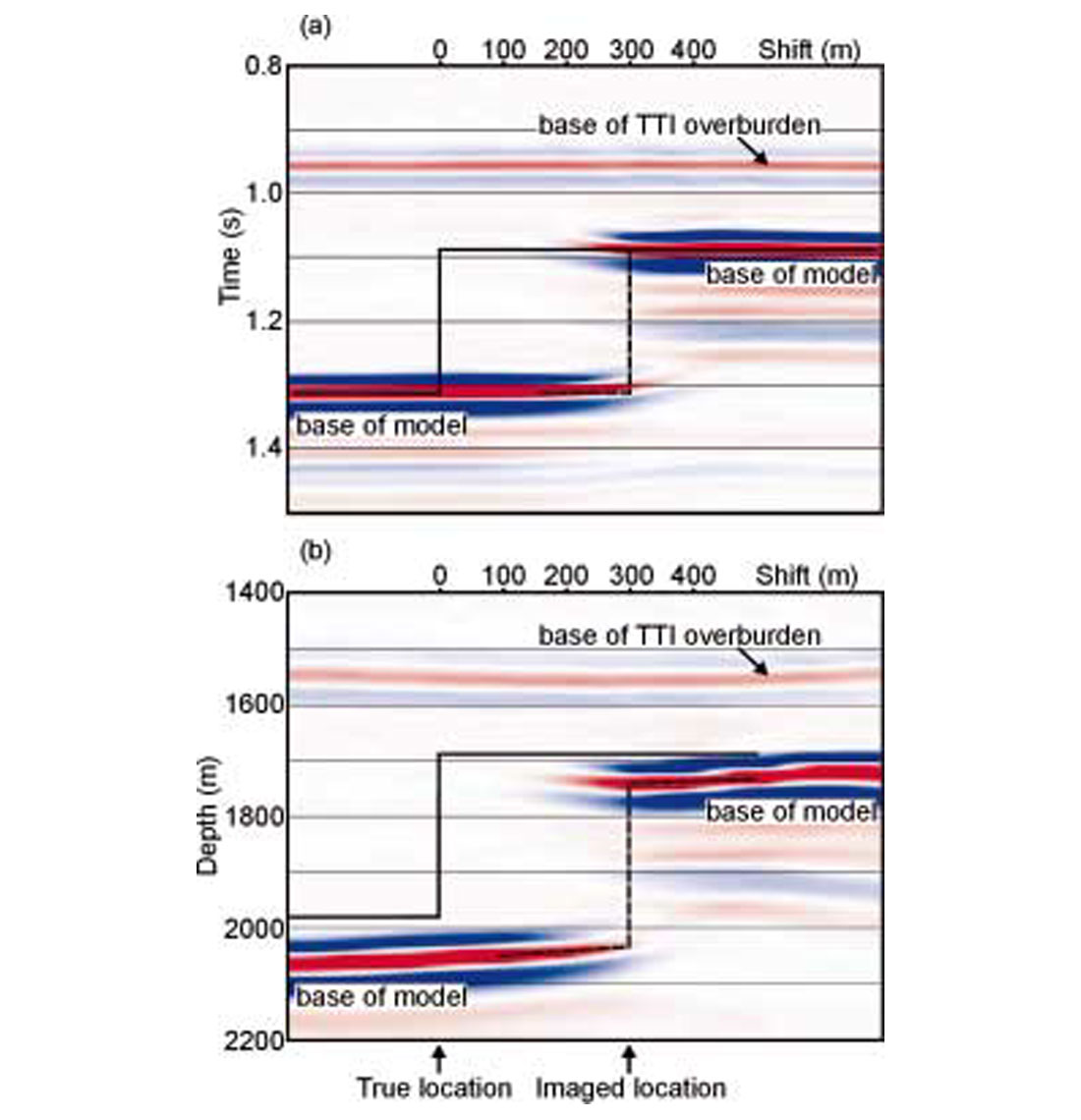
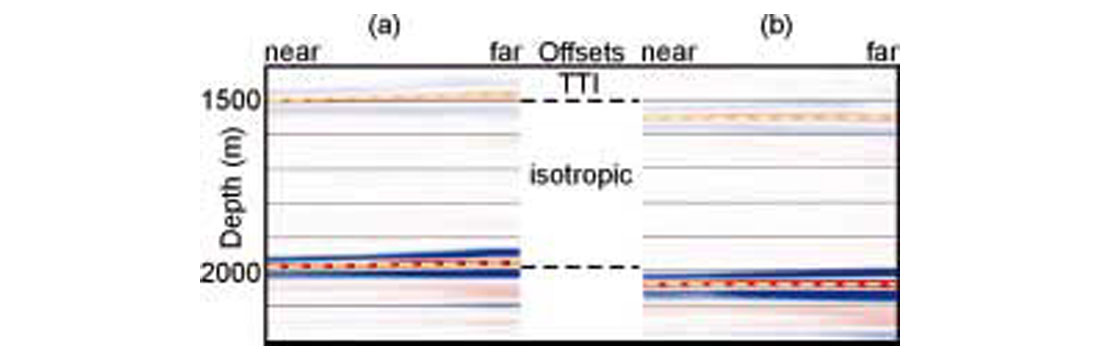
The data were processed using a velocity model obtained by standard semblance analysis. The prestack time migrated and prestack depth migrated sections (Figures 3a and 3b, respectively) both reveal an imaged step that is shifted 300 m updip from the true location. On the depth section, the depth of the base of the TTI layer is about 50 m too deep. The isotropic depth migration velocity analysis software used here works on the assumption that the correct velocity field produces consistent depth images in the common-offset domain. Residual moveout of reflectors in a common reflection point (CRP) gather is assumed to be indicative of an incorrect velocity field and that the correct velocity is the one which flattens the events in these gathers. Prestack depth migrated gathers obtained using a constant velocity of 3145 m/s in the anisotropic section have the correct depth on the near offsets but show residual moveout with offset (Figure 4a). A velocity of 3250 m/s in the anisotropic section flattens the CRP gathers (Figure 4b) but the depths obtained in these flattened gathers are too deep by 50 m (about 3% of the true depth).

3-D survey

The physical model created for the 3-D seismic survey (Figure 5) consists of a TTI overburden, dipping at 45° in the 1-3 plane, overlying a square target, which is cut into the base of an isotropic slab. Five source lines, each 2500 m long, were set out parallel to the dip direction and six receiver lines, each 2000 m long, were laid orthogonal to the source lines. Source and receiver station spacing was chosen to be 50 m so that the CDP bin size would be 25 m x 25 m. The nearest offset is constrained to 250 m by the physical size of the transducers while the farthest offset over the centre of the survey is 3200 m.

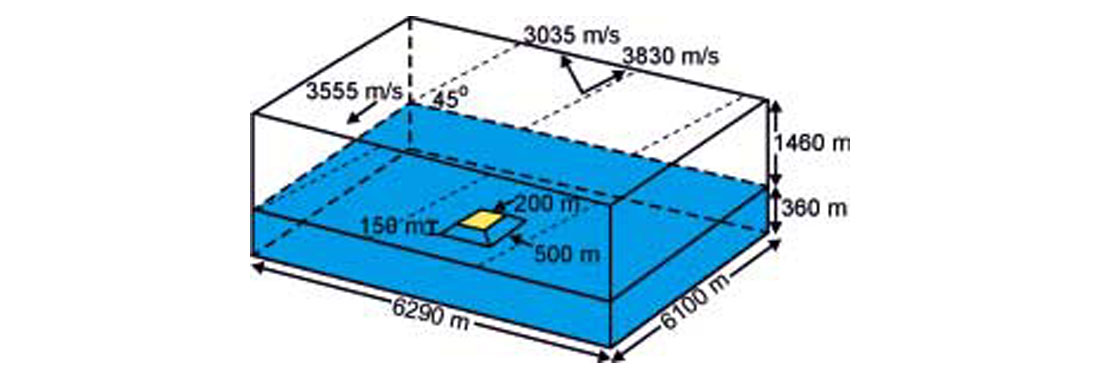
The data were processed using isotropic stacking velocities derived from standard 3-D semblance velocity analysis. After 3-D poststack time and depth migrations, which were the only 3-D migrations available to us at this time, the data were interpreted using Seis-X.

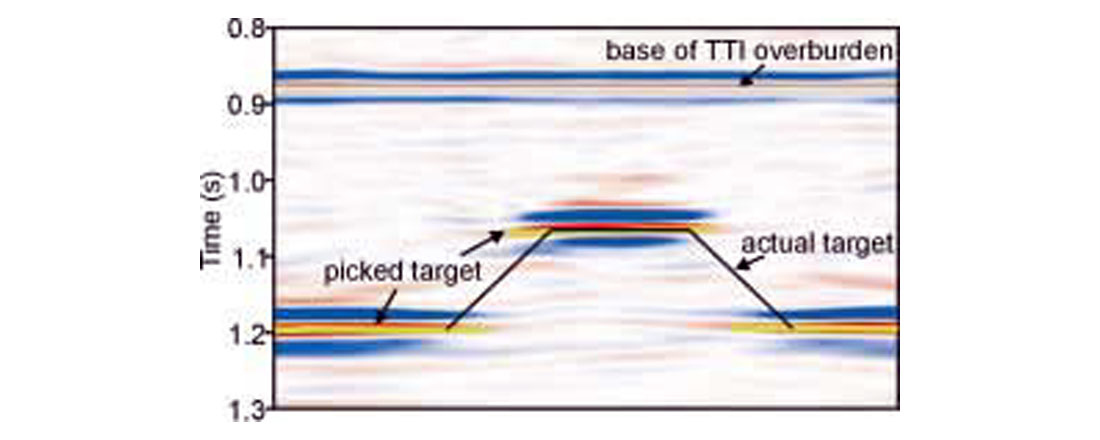
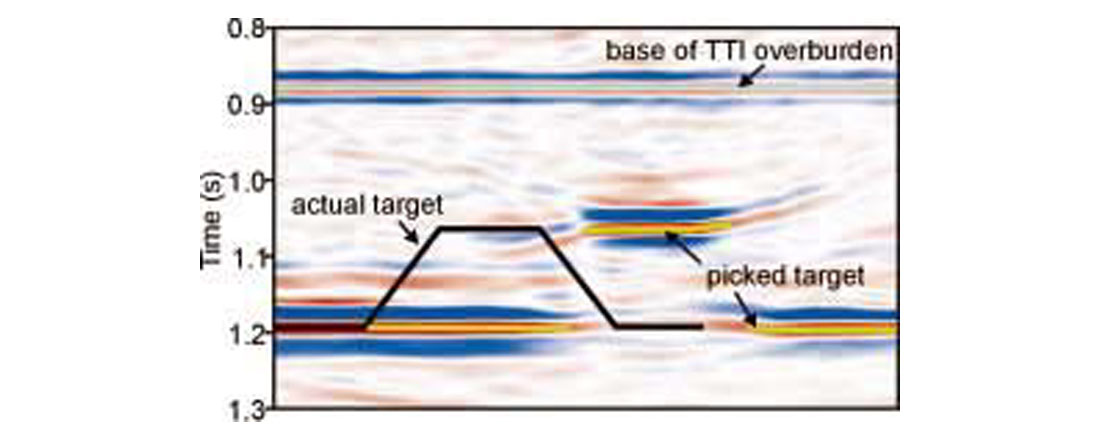
A strike line through the centre of the imaged target (Figure 6) shows no lateral displacement of the imaged target but the 45° sides of the target are not imaged. However, the top of the target is focussed nicely. On a dip line through the centre of the survey (Figure 7) the target is shifted 325 m towards the updip direction. To the sides of the top of the target (at about 1.05 s) the character of the data varies between the updip and downdip directions.

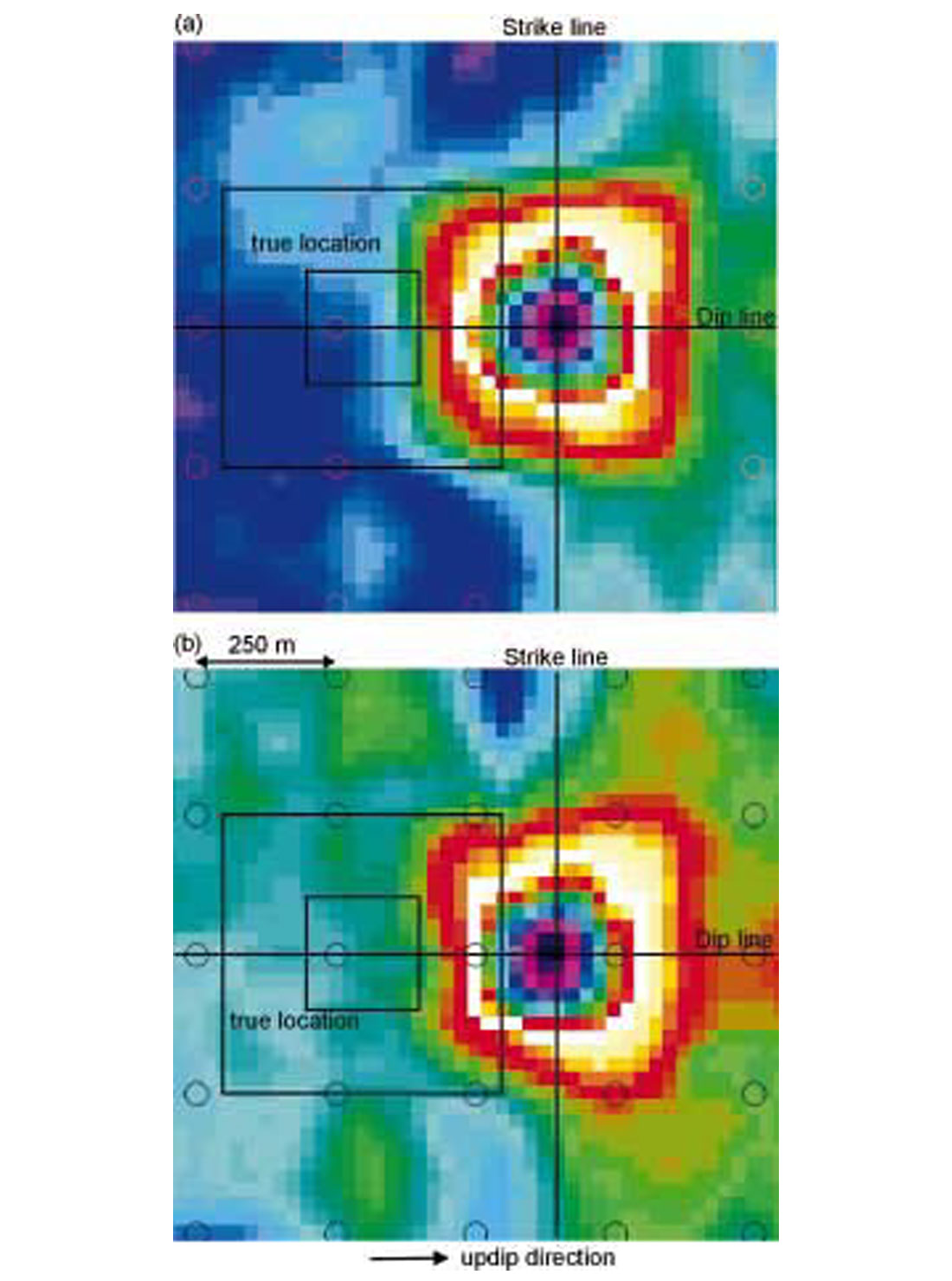
Amplitudes of the target horizon picked on the time and depth poststack migrated data are mapped in Figure 8. High amplitudes are represented by purple and low amplitudes by yellow and the true locations of the inner and outer squares of the target and the locations of the displayed lines are annotated. The true square shape of the target is not imaged exactly on this map. The target appears to be elongated a little on the updip side. Most significant is the shift of the target 325 m updip from the true location.

Discussion

These physical modeling experiments demonstrate the magnitude of the image mispositioning that could be incurred by assuming velocity isotropy when velocity anisotropy is present in the overburden. The lateral shift of the imaged targets in the updip direction is significant and on field data could lead to a serious mispositioning of well locations. These cases illustrate clearly the need for anisotropic depth migration. Development of 3-D anisotropic depth migration code is in progress.

Acknowledgements

We gratefully acknowledge the sponsors of the Fold-Fault Research Project, Eric Gallant of the CREWES Project, Landmark Graphics and Paradigm Geophysical.