A Question from the December (2008) luncheon…

At the CSEG luncheon of December 15, 2008, Jon Downton and I delivered a talk in which we evaluated the effectiveness of an interpolation process applied prior to pre-stack imaging. The focus of this analysis was the ability of the interpolator to minimize migration noise caused be geometric irregularities on land 3D seismic data. We were especially concerned with understanding how minimizing migration noise would aid in the AVO analysis of the pre-stack imaged gathers. In the course of this work, we created various versions of the data- with and without the interpolation- and observed differences in stacks, gathers, and AVO attribute maps. Objective measures of the accuracy improvements of the AVO analysis were the crux of this effort. At the conclusion of the talk, we were asked an interesting question by Norm Kalmanovich. Norm had observed that the stacked results of our interpolated pre-stack migrations were cleaner relative to the same product that lacked the interpolation. Norm was impressed with the difference, and suggested that perhaps we should comment more on the interpolation process’s ability to improve stack sections. Norm was particularly concerned about very noisy datasets with minimal signal. Norm’s observation is correct, but it is worthwhile to answer his query in more detail. In this response, I will talk about what kind of noise is removed, put forward a perspective on relative improvements that interpolation may yield depending on the product being observed, and also refer to a previous work that examined Norm’s question in greater detail.

The observation

Norm’s question comes from his astute observation of a stack comparison from the luncheon talk. Figure 1, below, is taken from that talk. This comparison was created to illustrate that the interpolation did not cause measure a b l e smearing of structural features on the imaged stack. A thrust fault was highlighted. Norm noted that the noise level was also considerably better on the interpolated data.

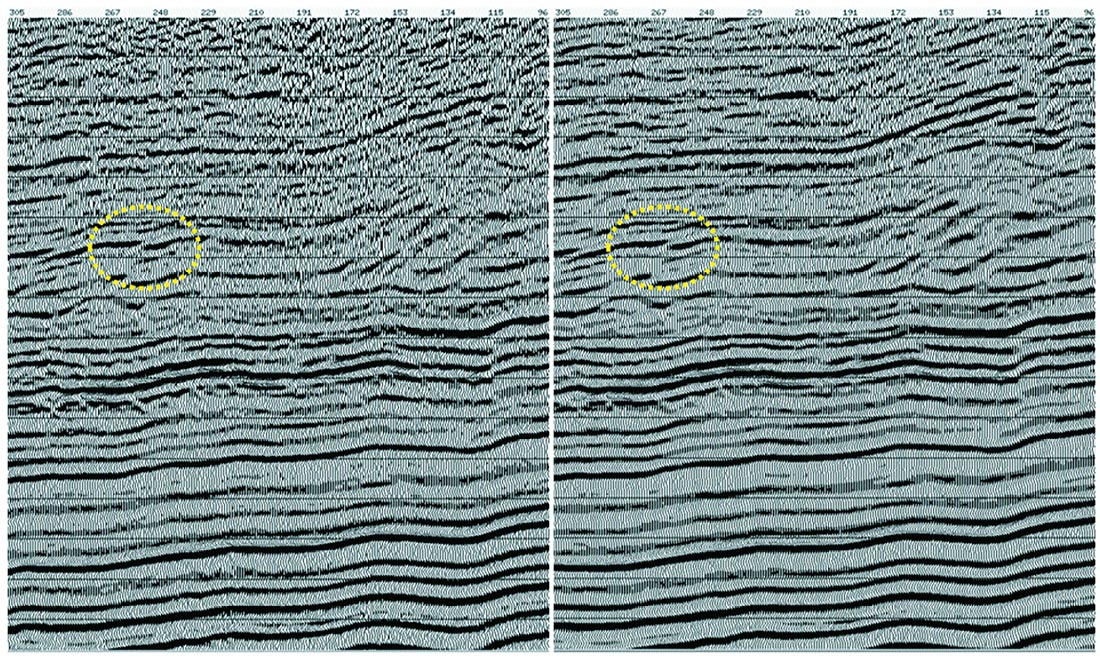
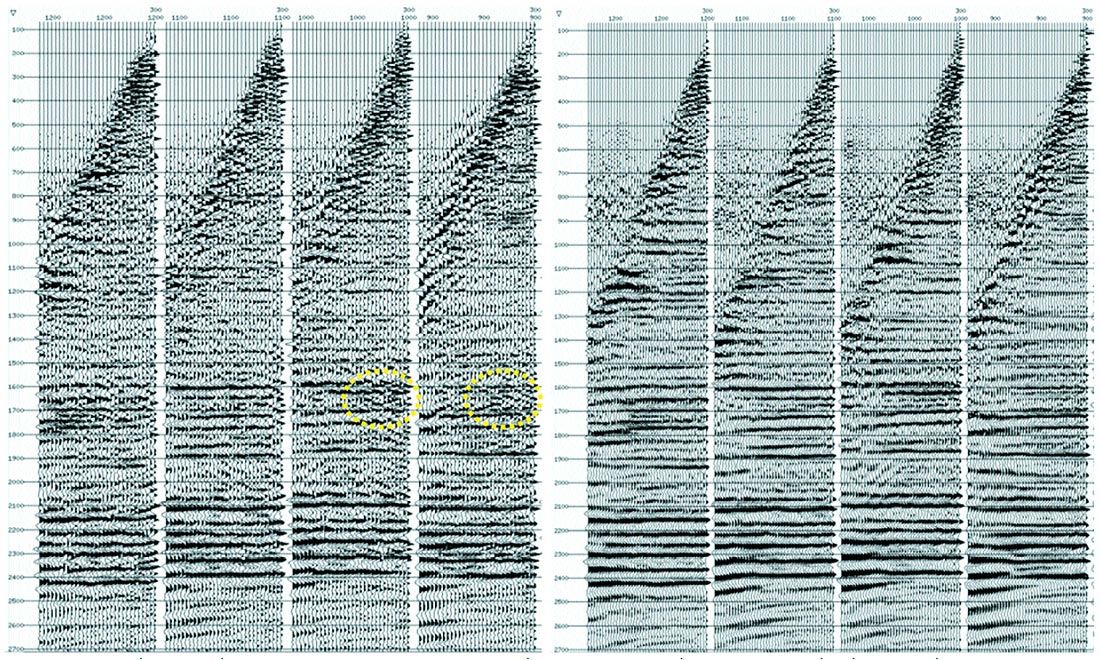
The differences in noise level illustrated in Figure 1 should also be compared to the differences in noise level observed in single fold pre-stack migrated gathers. This gather comparison, again taken from the luncheon, is illustrated in Figure 2. The differences in data quality are demonstrably greater in the gather comparison relative to the stack comparison. Figure 2, below, is arguably the most important slide from the luncheon talk, as it is possible to see what appear to be migration “smiles” in the uninterpolated gathers, a phenomena we predicted we would minimize with the interpolation process. This phenomena has been predicted and illustrated by others, including Cary in 2007, who showed the effect on synthetics.

Noise from the 3D experiment and noise from processing

Seismic processing is often concerned with minimizing the various kinds of noise inherent in the seismic experiment. This noise may be random or coherent, and can be observed on the shots, gathers, and stacks of the data. Pre-stack interpolation techniques may minimize some of the inherent seismic noise in a variety of ways including by adding to the fold of the data, and because the interpolator will only produce data that is coherent across its domains of operation. It is also true that there are many kinds of interpolators, each with different characteristics in regards to how noise may be treated. The purpose of the interpolation process is not, however, the removal of noise inherent to the seismic experiment; it is to minimize the amount of noise created by the pre-stack migration process. This is why Cary in 2005 said that if there is a large difference (in a non-structured example) of the data with a pre-stack migration versus a post stack migration, then there may be a problem with the migration process itself. Cary was referring to his Common Offset vector (COV) technique of regularizing pre-stack data, a method of much current interest.

Let us refer to Figure 2 (above) again. The migration artifacts in the uninterpolated gathers are a phenomena created by the migration process, that the interpolation or interpolation-like (regularizing) process is meant to reduce. This difference is not principally caused by a reduction in noise by the interpolation process itself, but rather by what the migration does afterwards. This point harkens to observations made by Mike Perz (personal communication) where he asked why more pre-stack migrated results do not look as good as post stack migrations. Mike was lamenting the fact that 3D pre-stack migration imposes more stringent sampling requirements on the input data relative to poststack migration and consequently the 3D PSTM stacks are often disappointing in how they suffer from migration noise. In 2008 Perz and Zheng illustrated that they could improve these migration artefacts in pre-stack migrations through interpolation. Mike's work is supportive of our thesis here, and speaks directly to Norm's question about stack data. By providing a more regularly sampled 3D dataset, interpolation (or methods with similar aims) will likely improve the pre-stack migrated stacked results of most land 3D seismic surveys. This argument assumes that land 3D seismic is typically shot coarsely and irregularly, which is almost always true. In 2005, Hunt et al gave a paper at the CSEG Convention discussing this. A version of that abstract is here.

Perspective on relative value from interpolation

If we accept that interpolation primarily minimizes noise created in the migration, then it might be possible to rank the importance of interpolation by the domains of observation we might be interested in, from most important to least:

1. Azimuthal analysis (AVAz)
2. AVO analysis
3. Wave Equation migration
4. Pre-stack migrated stack
5. Post-stack migrated stack

Azimuthal analysis is ranked highest because it requires an azimuth persevering migration, and its sampling will be the poorest. AVO analysis requires Pre-stack migrated gathers, and is not well sampled. The wave equation migrations require a regular geometry, so processes that enable that are critical to its use. The pre-stack migrated stack is less important that the others because much of the migration noise will be cancelled by the power of stacking. The post stack migrated product will be improved minimally. Typically this product will not have any migration noise, except across gaps in the data, and will only benefit from having higher fold.

It should also be mentioned that the interpolation process must honor the type of data being looked at. That is, if the data is being used for azimuthal analysis, the interpolator has to honor the azimuthal information in the data. If we are only stacking the data, there is less explicit need for the interpolator to honor azimuthal information. There are currently many kinds of interpolators available in industry, and a significant effort is being made to produce higher dimensional products so that AVO and AVAz may be considered. In the luncheon talk we discussed how, when optimally constrained, these higher dimensional interpolators sometimes benefitted from the additional dimensionality by being able to interpolate over greater distances as compared to simpler lower dimensional interpolators. Mike Perz and Sterwart Trickett made similar observations about this potential advantage of their higher dimensional interpolators in personal communications. Interpolation is a complex operation which changes based on the details of the implementation, the (algorithmic) engine running it, and constraints applied- all of which make sweeping generalizations on the subject dangerous. Despite the seriousness of that warning, it is also difficult to ignore the notion that the higher dimensional or more sophisticated new interpolators that include offset and azimuth may have the potential to be more advantageous in general, rather than only for projects that may undergo AVO or AVAz. Perhaps further work will be published in the future to explore some of these possibilities.

Acknowledgements

Perz, M., personal communication.

Trickett, S., personal communication.